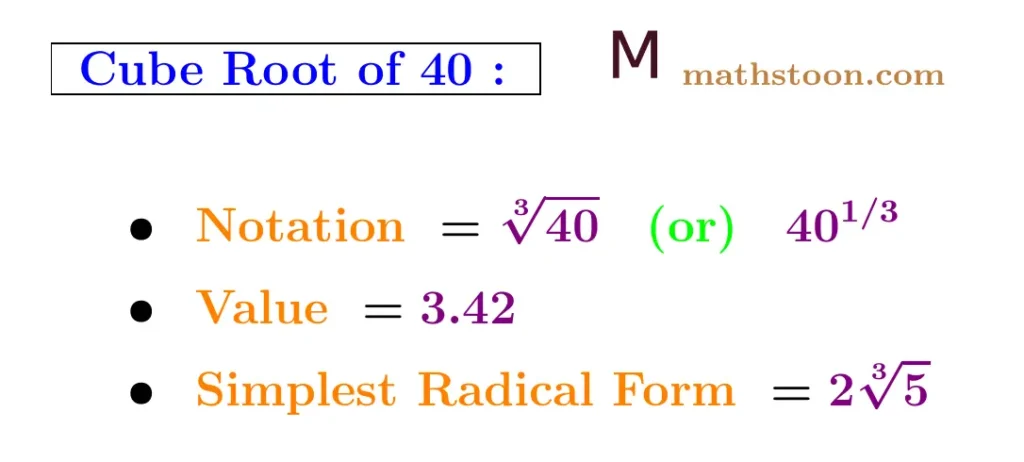
The cube root of 40 in simplified radical form is equal to 2∛5. The value of cube root of 40 is 3.42 corrected up to two decimal places. Note that a number m is called a cube root of 40 if m3 = 40. So it is a root of the cubic equation x3=40, so there are three cube roots of 40.
Some facts are listed below:
- Cube root of 40 in simplified radical form is 2∛5.
- ∛40 = 2∛5.
- 40 is not a prefect cube number.
- 401/3 is the exponential for of the cube root of 40.
- Value of cube root of 40 is 3.4199 (in decimal form) corrected up to 4 decimal places.
- cube of 40: 40×40×40 = 64000.
Table of Contents
What is the Cube Root of 40?
To find the cube root of 40, we will express the number 40 as a product of numbers at least one of them will be perfect cubes. We can write 40 as follows:
40 = 8×5.
Here 8 is a perfect cube as 8 = 23.
Taking cube root on both sides, we obtain that
| $\sqrt[3]{40} = \sqrt[3]{8 \times 5}$ ⇒ $\sqrt[3]{40} = \sqrt[3]{8} \times \sqrt[3]{5}$ using the rule: ∛ab = ∛a ∛b. ⇒ $\sqrt[3]{40} = 2 \times \sqrt[3]{5}$. |
That is, ∛40 = 2∛5.
So the cube root of 40 in its simplified radical form is equal to 2∛5.
By Prime Factorization
The prime factorization of 40 is given as follows:
40 = 2 × 2 × 2 × 5.
So $\sqrt[3]{40} = \sqrt[3]{2 \times 2 \times 2 \times 5}$
Make a grouping of 3 identical numbers, so we obtain that
| $\sqrt[3]{40} = \sqrt[3]{2 \times 2 \times 2} \times \sqrt[3]{5}$ ⇒ ∛40 = 2 × ∛5 ⇒ ∛40 = 2∛5. |
So the cube root of 40 by prime factorization method is equal to 2∛5, and it is its simplest radical form.
More cube roots:
Value of Cube Root of 40
As we know that cube root of 40 is given by ∛40 = 2∛5, and the value of ∛5 is 1.71 approximately, so we obtain that
∛40 = 2 × 1.71 = 3.42.
Therefore, 3.42 is the value of cube root of 40.
Also Read: cube root of 81
Is 40 a Perfect Cube
Answer: Note that 40 will be a prefect cube number only if its cube root is a whole number. We know that ∛40 = 2∛5 is not a whole number. This proves that the number 40 is not a perfect cube.
Is Cube Root of 40 Rational
Answer: The cube root of 40 is 2∛5, and the number ∛5 is irrational. Therefore, we conclude that cube root of 40 is not a rational number.
FAQs
Answer: The cube root of 40 in its simplest radical form is equal to 2∛5.
Answer: As ∛40 = 2∛5 is not an integer, 40 is not a perfect cube number.
This article is written by Dr. T, an expert in Mathematics (PhD). On Mathstoon.com you will find Maths from very basic level to advanced level. Thanks for visiting.