The derivative of fourth foot of x is equal to 1/(4x3/4). Fourth root of x is denoted by ∜x = x1/4, so its derivative formula is given by
$\dfrac{d}{dx}$(∜x) = $\dfrac{1}{4x^{3/4}}.$
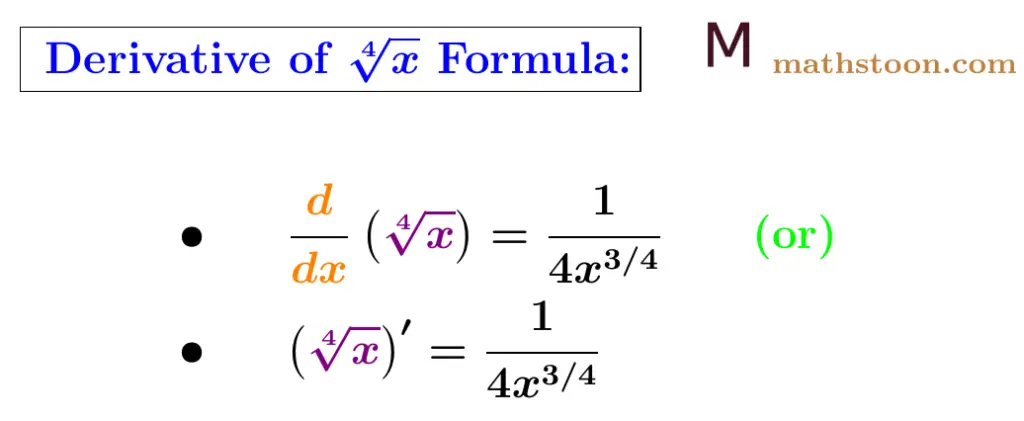
In this article, we will learn how to differentiate fourth root of x with respect to x by the following methods:
- Power rule of derivatives
- Substitution method
- First principle of differentiation
- Logarithmic differentiation.
Table of Contents
By Power Rule
As the fourth root of x is expressed as ∜x = x1/4, its derivative can be easily computed by the power rule of differentiation. This rule says that the derivative of xn is equal to nxn-1, that is,
d/dx (xn) = nxn-1.
Put n=1/4.
So we obtain that
| $\dfrac{d}{dx}$(∜x) = $\dfrac{d}{dx}(x^{1/4})$ ⇒ $\dfrac{d}{dx}$(∜x) = $\dfrac{1}{4}x^{\frac{1}{4}-1}$ ⇒ $\dfrac{d}{dx}$(∜x) = $\dfrac{1}{4x^{3/4}}$. |
So the derivative of fourth root of x by power rule is equal to 1/(4x3/4).
ALTERNATIVE METHOD: To find the derivative of fourth root of x, let us substitute $y=\sqrt[4]{x}.$. This implies that
y4=x.
Differentiate both sides with respect to x. So we get that
$4y^3 \dfrac{dy}{dx}=1$
⇒ $\dfrac{dy}{dx}=\dfrac{1}{4y^3}$
⇒ $\dfrac{dy}{dx}=\dfrac{1}{4(\sqrt[4]{x})^3}$ as we have $[\because y=\sqrt[4]{x}]$
⇒ $\dfrac{dy}{dx}=\dfrac{1}{4x^{3/4}}$
This proves that the derivative of fourth root of x is 1/(4x3/4), and we get this by the substitution method.
Also Read: Derivative of cube root of x
Derivative of Fourth Root of x by First Principle
By first principle, the derivative of f(x) is given by the following limit formula:
d/dx (f(x)) = limh→0 $\dfrac{f(x+h)-f(x)}{h}$.
Let f(x) = ∜x. Note that
f(x) = x1/4 and f(x+h) = (x+h)1/4.
Then by first principle,
| $\dfrac{d}{dx}$(∜x) = limh→0 $\dfrac{(x+h)^{\frac{1}{4}} – x^{\frac{1}{4}}}{h}$ |
Put x+h = z. So z→x as h→0. Also, h=z-x.
Therefore,
| So $\dfrac{d}{dx}$(∜x) = limz→x $\dfrac{z^{\frac{1}{4}} – x^{\frac{1}{4}}}{z-x}$ = $\dfrac{1}{4}x^{\frac{1}{4}-1}$. Here we have used the formula limx→a (xn-an)/(x-a) = nan-1. |
Hence, $\dfrac{d}{dx}$(∜x) = $\dfrac{1}{4x^{3/4}}$.
Therefore, the derivative of fourth root of x is equal to 1/(4x3/4) and this is obtained by the first principle of differentiation.
Related Topics:
By Logarithmic Differentiation
Let y = ∜x = x1/4.
Taking natural logarithms on both sides, we get that
ln y = 1/4 ln x
Differentiating with respect to x,
$\dfrac{1}{y} \dfrac{dy}{dx}=\dfrac{1}{4} \cdot \dfrac{1}{x}$
⇒ $\dfrac{dy}{dx}=\dfrac{y}{4x} = \dfrac{x^{1/4}}{4x} = = \dfrac{1}{4x^{3/4}}$.
So by logarithmic differentiation, the derivative of fourth root of x is equal to 1/(4x3/4).
Solved Problems
Question 1: Find the derivative of fourth root of a where a is a constant. That is, find d/dx(∜a).
Answer:
Note that the fourth root of $a$ is a constant. We know that the derivative of a constant is zero. So we obtain that
d/dx(∜a) = 0.
⇒ d/dx(a1/4) = 0.
Remark: Put a=1. So the derivative of fourth root of 1 with respect to x is equal to 0, that is, d/dx(∜1) = 0.
Question 2: Find the derivative of fourth root of x+1, i.e, find d/dx(∜(x+1)).
Answer:
Put t=x+2.
So dt/dx = 1.
Now by the chain rule of derivatives,
$\dfrac{d}{dx}$ (∜(x+1)) = $\dfrac{d}{dt}$ (∜t) × $\dfrac{dt}{dx}$
= 1/(4t3/4) × 1
= 1/{4(x+1)3/4}.
So the derivative of (x+1)1/4, that is, fourth root of x+1 is equal to 1/{4(x+1)3/4}.
FAQs
Answer: The derivative of x1/4 (fourth root of x) is equal to 1/(4x3/4).
Answer: d/dx(∜x) = 1/(4x3/4).
This article is written by Dr. T, an expert in Mathematics (PhD). On Mathstoon.com you will find Maths from very basic level to advanced level. Thanks for visiting.