Derivative of mod x: Formula, Proof | Mod x Derivative

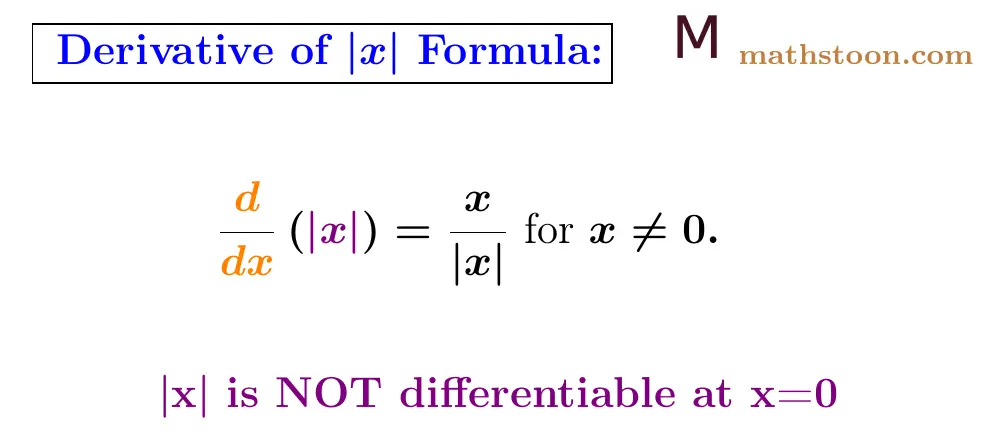
The derivative of |x| (mod x) is equal to x/|x| where x is non-zero. The function |x| is known as the absolute value of x. So the derivative of absolute value of x is given by

$\dfrac{d}{dx}(|x|) = \dfrac{x}{|x|}$, provided that x ≠ 0.

Before we prove this formula, let us first recall the definition of |x|. Modulus of x is defined as follows:

|x| = x if x ≥ 0 $\quad$= -x if x < 0.

What is the Derivative of Mod x?

Answer: The derivative of mod x is equal to $\dfrac{d}{dx}(|x|) = \dfrac{x}{|x|}$ when x is non-zero. At x=0, the derivative of mod x doest not exist.

Proof of Derivative of mod x

We prove this formula case by case.

Case1: Suppose x > 0.

By the definition of mod x, we know that |x| = x for x > 0.

Differentiating,

$\dfrac{d}{dx}|x| = \dfrac{d}{dx}(x)$

⇒ $\dfrac{d}{dx}|x| = 1$.

Case2: Suppose x < 0.

By definition, we have |x| = -x for x < 0.

Differentiating,

$\dfrac{d}{dx}|x| = \dfrac{d}{dx}(-x)$

⇒ $\dfrac{d}{dx}|x| = -1$.

So we have shown that

$\dfrac{d}{dx}(|x|) = \begin{cases} 1, & \text{ if } x > 0 \\ -1, & \text{ if } x < 0. \end{cases}$

Rewriting the above we obtain that the derivative of mod x is equal to

$\dfrac{d}{dx}(|x|) = \dfrac{x}{|x|}$ for x non-zero.

Case3: Let x=0.

We will show that the derivative of |x| does not exist at x=0.

Let f(x) = |x|.

The left-hand limit = lim_h→0- $\dfrac{f(h)-f(0)}{h}$

= lim_h→0- $\dfrac{|h|-0}{h}$

= lim_h→0- $\dfrac{-h-0}{h}$

= -1.

The right-hand limit = lim_h→0+ $\dfrac{f(h)-f(0)}{h}$

= lim_h→0+ $\dfrac{|h|-0}{h}$

= lim_h→0+ $\dfrac{h-0}{h}$

= 1.

As the left-hand and the right hand limit are not equal, so the limit lim_h→0 $\dfrac{f(h)-f(0)}{h}$ does not exist. Therefore, mod x is not differentiable at x=0.

Also Read: Integration of |x|

Derivative of root x 

Derivative of x√x

General Situation

The modulus of x-a (a is a constant), that is, |x-a| is differentiable at every point except x=a. The function |x-a| is defined as follows:

|x-a| = x-a if x ≥ a $\quad \quad$= -(x-a) if x < a.

Differentiability of |x-a| at x=a

Let f(x) = |x-a|. Assume a>0.

The left-hand limit =

lim_h→0- $\dfrac{f(a+h)-f(a)}{h}$ = lim_h→0- $\dfrac{|h|-0}{h}$ = lim_h→0- $\dfrac{-h-0}{h}$ = -1.

The right-hand limit =

lim_h→0+ $\dfrac{f(a+h)-f(a)}{h}$ = lim_h→0+ $\dfrac{|h|-0}{h}$ = lim_h→0+ $\dfrac{h-0}{h}$ = 1.

As the above two limits are unequal, we conclude that modulus of x-a is not differentiable at x=a.

Read Also: Derivative of cube root of x

FAQs