SN Dey Class 11 Chapter Solutions. Short answers on compound angles. Compound Angles SATQ. Solutions of Trigonometric Ratios of Compound Angles Short Answer Type Questions.
Ex 1(i): Prove that sin2α + sin2(120°-α) + sin2(120°+α) = $\frac{3}{2}$
Solution:
Ex 1(ii): Prove that sin2($\frac{\pi}{8}+\frac{\theta}{2}$) – sin2($\frac{\pi}{8}-\frac{\theta}{2}$) = $\frac{1}{\sqrt{2}}$ sinθ
Solution:

Ex 1(iii): Prove that cos2A + cos2(A+$\frac{\pi}{3}$) + cos2(A-$\frac{\pi}{3}$) = $\frac{3}{2}$
Solution:
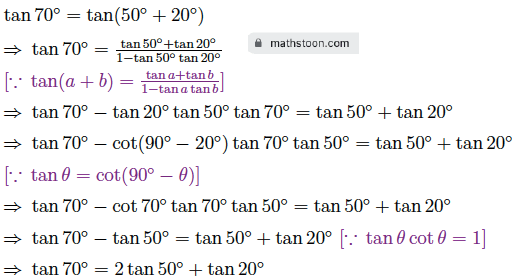
Ex 1(iv): Prove that tan70° = 2 tan50° + tan20°
Solution:
Ex 2(i): Simplify cosA sin(B-C) + cosB sin(C-A) + cosC sin(A-B)
Solution:
cosA sin(B-C) + cosB sin(C-A) + cosC sin(A-B)
= cosA (sinB cosC-cosB sinC) + cosB(sinC cosA – cosC sinA) + cosC(sinA cosB – cosA sinB)
= cosA sinB cosC – cosA cosB sinC + cosA cosB sinC – sinA cosB cosC + sinA cosB cosC – cosA sinB cosC
= 0
Ex 2(ii): Simplify $1+\frac{\sin(A-B)}{\cos A\cos B}$ $+\frac{\sin(B-C)}{\cos B\cos C}$ $+\frac{\sin(C-A)}{\cos C\cos A}$
Solution:
Ex 2(iii): Simplify tan($\frac{\pi}{4}+θ$) + tan($\frac{3\pi}{4}+θ$)
Solution:
Ex 2(iv): Simplify sin(B+C) sin(B−C) + sin(C+A) sin(C−A) + sin(A+B) sin(A−B)
Solution:
sin(B+C) sin(B-C) + sin(C+A) sin(C-A) + sin(A+B) sin(A-B)
= (sin2B – sin2C) + (sin2C – sin2A) + (sin2A – sin2B)
[∵ sin(α+β) sin(α-β) = sin2α – sin2β]
= 0
This article is written by Dr. T, an expert in Mathematics (PhD). On Mathstoon.com you will find Maths from very basic level to advanced level. Thanks for visiting.